Unit 15: Temperature, Heat, and Expansion
A given amount of distilled water reaches its minimum volume (or maximum density) at 4°C. Any deviation from this temperature causes the water to expand. After completing this unit, you should be able to explain this unique phenomenon and discuss its positive impact on marine life.
Learning Outcomes
By the end of this unit, you should be able to
- explain the notion of temperature.
- define heat and internal energy.
- discuss how heat is measured.
- define specific heat capacity.
- explain thermal expansion and give examples from everyday life.
- explain the unusual thermal expansion of water near its freezing point.
eText Material
Reading Assignment
Read the following sections in Chapter 15 of the eText:
- 15.1: Temperature
- 15.2: Heat
- 15.3: Specific Heat Capacity
- 15.4: The High Specific Heat Capacity of Water
- 15.5: Thermal Expansion
Supplementary learning resources are available on the Mastering Physics learning platform.
Additional Reading
Heat, Temperature, and Internal Energy
Even though heat and temperature are closely related, they are two distinct physical quantities. Heat flow describes a change in the internal energy content of a substance, whereas temperature is a measure of the average kinetic energy of the atoms (or molecules) in the substance. Quantitatively, heat is measured in joules (J) or calories (cal), whereas temperature is measured in degrees Celsius (°C), Kelvin (K), or degrees Fahrenheit (°F).
Internal energy is the total (kinetic plus potential) microscopic energy in an object. Heat does not necessarily flow from an object of higher internal energy content to an object of lower internal energy content. However, heat flows spontaneously from an object of higher temperature to an object of lower temperature until the temperature of both objects become equal.
Consider two blocks of steel at room temperature, say 20°C, where one block has a mass of 1 kg and the other is 2 kg. Suppose you supply enough heat (110 cal) to raise the temperature of the larger block by 0.5°C so its temperature becomes 20.5°C. If you supply the same amount of heat to the smaller block, it will reach a final temperature of 21°C. Note that although both blocks received equal amounts of heat, the temperature increase of the smaller block is double that of the larger block.
Specific Heat Capacity
Consider a block of steel and a block of sliver, each initially at room temperature (20°C) and each having a mass of 1 kg. If you supply 110 cal to the steel block, its temperature increases to 21°C, as mentioned earlier. However, the same amount of heat will raise the temperature of the silver block to 22°C. This is because steel has a greater specific heat capacity than silver.
The specific heat capacity of a substance is the quantity of heat required to raise the temperature of a unit mass of the substance by 1°C. For example, you need 1 cal (4.186 J) to increase the temperature of 1 g of water from 14.5°C to 15.5°C. Therefore, the specific heat capacity of water is equal to $1\,\text{cal}/{\text{g}\cdot\text{C}^\circ}$.
Temperature Scales
The first modern thermometers were designed by Daniel Gabriel Fahrenheit (1686–1736), a Dutch manufacturer of meteorological instruments, who experimented with using alcohol and mercury in thermometers. On his scale, the temperature of melting ice is 32°F and that of boiling water is 212°F. This scale, known as the Fahrenheit scale, is still used in some countries, including the United States.
The 180-degree difference between the freezing and boiling point of water was not aesthetically pleasing to many scientists, and they sought to establish a scale based on a difference of 100 degrees between the two fixed points. Anders Celsius (1701–1744), a Swedish astronomer, was the first to use such a scale, with the freezing temperature of water set at 0°C and the boiling temperature set at 100°C.
Scientists, however, prefer to use the absolute scale of temperature known as the Kelvin scale. Absolute zero on the Kelvin scale ($0\,\text{K} = -273^\circ\text{C}$) corresponds to the temperature at which all atomic and molecular motion comes to a halt.
Thermal Expansion
One of the earliest discoveries in the study of the thermal properties of matter was that most substances expand with the application of heat. This phenomenon is called thermal expansion. For example, when heated, a long, thin wire will have a noticeable increase in length that is proportional to its original length and to the increase in temperature. A solid object also expands in all three dimensions (i.e., swells in volume) as it becomes warmer.
You may have noticed that railroad tracks are not continuous: there are gaps between successive pieces of track to accommodate expected thermal expansion. Without the gaps, on a hot day, the tracks would experience a huge thermal stress and would buckle. Engineers must make allowances for thermal stresses when they are designing buildings, bridges, and, of course, railroad tracks.
The Ideal Gas Law
Suppose you put a certain amount of nitrogen gas in a cylinder with an adjustable piston. The piston, which is assumed to be airtight, can be moved to change the volume inside the cylinder. The gas exerts a pressure ($P$) on the walls of the cylinder and on the inside surface of the piston.
Suppose you push the piston so the volume of the container—and, therefore, the volume of the gas ($V$)—is now half of the original volume. Note that the cylinder still contains the same number of nitrogen molecules ($N$). If you make sure the temperature of the gas does not change during the procedure, you will find that the pressure exerted by the gas increases to twice the original pressure. This property—that pressure exerted by a gas is inversely proportional to its volume when the temperature is constant—is known as Boyle’s law.
Now suppose you increase of the temperature of the gas while holding the piston to maintain a constant volume. You will notice that the pressure exerted by the gas increases linearly with the increasing temperature ($T$), measured in kelvins. If you adjust the piston in a controlled way to ensure that the pressure remains constant, you will find that the volume increases linearly with temperature, which is known as Charles’s law.
An important relation that combines all the variables mentioned above is referred to as the ideal gas law, and it can be expressed in the following form: \begin{equation} P V = N k\, T \label{unit15_PVNKT} \end{equation} In this equation, $k$ is called the Boltzmann constant.
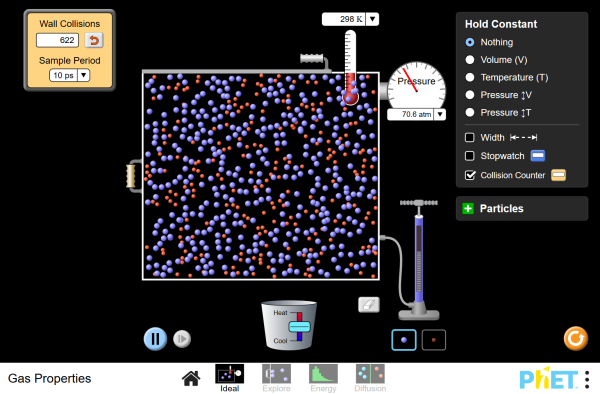
Simulation
Run the Gas Properties simulation to virtually pump gas molecules into a box and see what happens as you change the volume and add or remove heat.
Simulation by PhET Interactive Simulations, University of Colorado Boulder, licensed under CC-BY-4.0 (https://phet.colorado.edu)
Questions
The following questions are selected from the end of Chapter 15 of the eText. It is important to your learning that you try to answer each question independently before you read through the answer and explanation given.
For questions that ask you to explain, defend, or discuss your answer, the response revealed by the Answer button would earn you only partial marks on a quiz or exam in this course. Use the Answer to help you formulate a complete answer before you select the Explanation button to check your work.
Note that some questions have been adapted to suit the format of this course.
Chapter 15
Question 45
Which is greater: an increase in temperature of 1 Celsius degree or an increase of 1 Fahrenheit degree?
Answer
1 Celsius degree.
Diagram
Figure Q15.45
Explanation
On the Celsius scale, the temperature difference between the freezing and boiling points of water is equal to \begin{equation} 100 - 0 = 100^\circ\text{C.} \end{equation} On the Fahrenheit scale, the same temperature difference is equal to \begin{equation} 212 - 32 = 180^\circ\text{F.} \end{equation} So, as shown in Figure Q15.45, a change of 1 degree on the Celsius scale corresponds to a temperature difference of 1.8 (or 9⁄5) degrees on the Fahrenheit scale.
Chapter 15
Question 49
On average, which has more kinetic energy: a molecule in a gram of ice water or a molecule in a gram of steam? Defend your answer.
Answer
A molecule in a gram of steam has more kinetic energy.
Explanation
Temperature is a measure of the average molecular kinetic energy in a substance. Therefore, the average kinetic energy of a molecule in a gram of steam at 100°C is much greater than the kinetic energy of a molecule in a gram of ice water at 0°C.
Chapter 15
Question 59
A certain quantity of heat is supplied to both a kilogram of water and to a kilogram of iron. Which undergoes the greater change in temperature? Defend your answer.
Answer
The kilogram of iron undergoes a greater change in temperature.
Explanation
The specific heat capacity for water is $1\,\text{kcal}/\text{kg}\cdot\text{C}^\circ$, and for iron, it is $0.11\,\text{kcal}/\text{kg}\cdot\text{C}^\circ$. In other words, 1000 cal of heat are required to raise the temperature of 1 kg of water by 1°C, whereas only 110 cal are needed to increase the temperature of 1 kg of iron by 1°C. This also means that 1000 cal of heat supplied to 1 kg of iron will cause its temperature to increase by 9°C.
Chapter 15
Question 62
In times past, on a cold winter night, it was common to bring a hot object to bed with you. Which would keep you warmer through the cold night: a 10-kg iron brick or a 10-kg jug of hot water at the same temperature? Explain.
Answer
The jug of hot water would keep you warmer through the night.
Explanation
The specific heat capacity of iron is approximately one-tenth that of water. Therefore, water stores 10 times the thermal energy (or heat) contained in an equal mass of iron at the same temperature. More specifically, when a 10-kg iron brick cools down from 80°C to 0°C, it releases an amount of thermal energy calculated as follows: \begin{align} \text{Heat (iron)} &= \text{specific heat capacity}\times \text{mass}\times \text{temperature change} \nonumber\\[6pt] &= 0.11\,\text{kcal}/\text{kg}\cdot^\circ\!\text{C}\;\times\, 10\,\text{kg}\; \times\, 80^\circ\text{C} \nonumber\\[6pt] &= 88\,\text{kcal} \end{align} An equal mass of water that goes through the same temperature change delivers the amount of heat given below: \begin{align} \text{Heat (water)} &= \text{specific heat capacity}\times \text{mass}\times \text{temperature change} \nonumber\\[6pt] &= 1.0\,\text{kcal}/\text{kg}\cdot^\circ\!\text{C}\;\times\, 10\,\text{kg}\; \times\, 80^\circ\text{C} \nonumber\\[6pt] &= 800\,\text{kcal} \end{align}
Chapter 15
Question 67
Desert sand is very hot in the day and very cool at night. What does this indicate about its specific heat capacity?
Answer
It is relatively small.
Explanation
When compared with water, sand has relatively low specific heat capacity. Therefore, when equal masses of sand and water absorb the same amount of solar energy, sand experiences a greater increase in temperature. You can imagine sand to have a smaller energy tank that fills up more quickly, compared with that of water. The reverse situation explains the cooling effect when heat is radiated back during the night.
Chapter 15
Question 70
Would a bimetallic strip function if the two different metals had the same rates of expansion? Is it important that they expand at different rates? Explain.
Answer
No, it would not function. Yes, the two different metals must expand at different rates.
Explanation
Because of their different rates of thermal expansion, one of the metals in a functioning bimetallic strip becomes longer than the other metal when the temperature changes, which causes the strip to bend in the direction of the shorter metal. If the bimetallic strip consisted of two metals having the same rate of expansion, it would remain straight regardless of the temperature.
Chapter 15
Question 73
Why is it important that glass mirrors used in astronomical observatories be composed of glass with a low “coefficient of expansion”?
Answer
To increase the reliability of the astronomical instruments across different temperature conditions.
Explanation
The changes in temperature between daytime and nighttime and between summer and winter can alter the shape of glass mirrors and affect the precision of astronomical observations. Using glass with a low coefficient of thermal expansion helps minimize mirror distortions so more-accurate observations can be made at any time all year round.
Chapter 15
Question 77
Why are light bulbs typically made of very thin glass?
Answer
To ensure uniform heating of the glass.
Explanation
When the bulb is turned on, the glass heats up very quickly. With thin glass, both the inner and outer sides heat almost simultaneously, which causes the glass to expand uniformly. If the bulb were made of thick glass, it would experience nonuniform expansions and contractions, which could cause the glass to break.
Chapter 15
Question 92
Gas is sold by volume. Would you or the gas company gain by having gas warmed before it passed through your gas meter?
Answer
The gas company would gain.
Explanation
A gas meter measures the volume (or liters) of gas that passes through the device. If the gas was warmed, its volume would increase, causing the meter to overestimate the amount (or mass) of gas delivered to your house.
Chapter 15
Question 95
If you drop a hot rock into a pail of water, the temperatures of the rock and the water will change until both are equal. The rock will cool and the water will warm. Does this hold true if the hot rock is dropped into the Atlantic Ocean? Discuss.
Answer
Yes, the rock will cool down and the water in the Atlantic Ocean will warm up until the two temperatures are equal. However, due to the immense amount of water in the ocean, the increase in its temperature will be practically undetectable.
Exercises
Spend some time completing the following exercises to test your understanding of the main concepts in Chapter 15 and increase your efficiency in answering exam questions.
End-of-Chapter Practice Questions
Answer questions 1, 7, 13, 15, 17, 19, 25, 35, 51, 53, 61, 65, 75, 79, 91, 93, and 101 in Chapter 15 of the eText. If you require assistance, please contact your tutor. The answers are provided at the end of the eText.