Unit 9: Gravity
Did you know that if it were not for the force of static friction, two pickup trucks parked side by side in a parking lot would slowly slide toward each other and stick together in less than an hour? After completing this unit, you should be able to use Newton’s law of universal gravitation to explain this phenomenon.
Learning Outcomes
By the end of this unit, you should be able to
- state Newton’s law of universal gravitation and write its mathematical representation.
- define the terms weight and weightlessness.
- explain how ocean, atmosphere, Earth, and Moon tides occur.
- define the term gravitational field and discuss how its intensity varies outside and inside a planet.
- explain how a black hole is formed.
eText Material
Reading Assignment
Read the following sections in Chapter 9 of the eText:
- 9.1: The Universal Law of Gravity
- 9.2: The Universal Gravitational Constant, $G$
- 9.3: Gravity and Distance: The Inverse-Square Law
- 9.4: Weight and Weightlessness
- 9.5: Ocean Tides
- 9.6: Gravitational Fields
- 9.7: Black Holes
- 9.8: Universal Gravitation
Supplementary learning resources are available on the Mastering Physics learning platform.
Additional Reading
Law of Universal Gravitation
Newton’s discovery of the law of universal gravitation provides an interesting insight into the world of scientific activity. Observations, theories, and ideas from various scientists and philosophers float around for centuries as pieces of a puzzle until a scientist arrives at the right time in history, puts the various pieces together, and makes the picture complete—and a great theory is born. Of course, Newton did not suddenly stumble on the law of gravitation one fine morning! Ideas regarding attractive forces between different bodies had existed from the early days of scientific reasoning. Several scientists, mathematicians, and philosophers before and during Newton’s time had ideas that could have led to the law of gravitation.
Newton’s law of universal gravitation, which is considered the crowning achievement of his work, states:
Any two particles attract each other with a force that is proportional to the product of their masses and inversely proportional to the square of the distance between them.
Figure 9.1: Attractive forces of gravity between two spherical bodies of masses $m_1$ and $m_2$, separated by a distance $r$.
If two masses $m_1$ and $m_2$ are separated by a distance $r$, then each mass exerts an attractive force $F$ on the other along the line joining them. Note that the forces shown in Figure 9.1 form an action–reaction pair and the magnitude of each force is given by the equation \begin{equation} F = G\, \frac{m_1\, m_2}{r^2} \end{equation} where the value of the universal gravitational constant is determined experimentally to be \begin{equation} G = 6.67 \times 10^{-11}\, \text{N}\cdot\text{m}^2/\text{kg}^2 \end{equation}
For example, Earth exerts a gravitational force on the Moon equal to \begin{equation} G \times \frac{(5.98\times 10^{24}\,\text{kg}) (7.36\times 10^{22}\,\text{kg})}{(3.84\times 10^8\,\text{m})^2} = 2.0\times 10^{20}\, \text{N} \end{equation} and the Moon exerts an equal force on Earth in the opposite direction. The distance $r = 3.84\times 10^8\,\text{m}$ is the distance between the center of Earth and the center of the Moon.
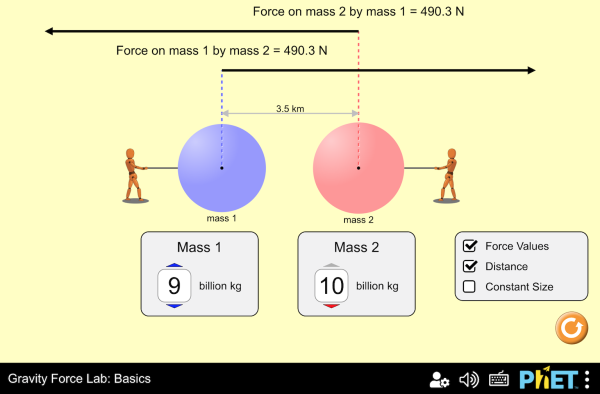
Simulation
Run the Gravity Force Lab simulation to discover the factors that affect gravitational attraction and determine how adjusting these factors changes the gravitational force.
Simulation by PhET Interactive Simulations, University of Colorado Boulder, licensed under CC-BY-4.0 (https://phet.colorado.edu)
Apparent Weight
Normally, the weight of a person standing on a bathroom scale is indicated by the scale’s reading. However, this is not always true! There are special circumstances when a properly functioning scale overestimates or underestimates someone’s weight. This is because the scale measures the normal force exerted by the scale on the person’s feet, which could be different from the actual weight.
Consider a person standing on a bathroom scale inside an elevator that is either at rest or moving at a constant speed (i.e., $a = 0$). The person experiences two forces in this case: the downward weight $F_g$ and the upward normal force $N$ (see the “Constant speed” image in Figure 9.2). Since the system does not accelerate, the net force on the person must be equal to zero. According to Newton’s second law, \begin{align} N - F_g &= F_\text{net} \nonumber\\[6pt] &= 0 \nonumber\\[6pt] \Rightarrow\quad N &= mg \end{align} So, in this case, the bathroom scale measures the person’s true weight ($F_g = mg$).
Figure 9.2: True and apparent weights of a person standing on a bathroom scale inside a moving elevator.
During the acceleration phase of the elevator’s motion (i.e., $a \neq 0$), the person experiences a net vertical force, which is given as
\begin{align} N - F_g &= F_\text{net} \nonumber\\[6pt] &= m a \nonumber\\[6pt] \Rightarrow\quad N &= F_g + m a \label{ch05_Nwma} \end{align}
As mentioned earlier, the bathroom scale is sensitive to the normal force $N$, which can be referred to as the apparent weight. In Equation 9.5, you see that the apparent weight is greater than the true weight when the acceleration is directed upward ($a \gt 0$). This is why a person feels heavier than normal at the beginning of an upward trip or near the end of a downward trip in an elevator. On the other hand, when the elevator slows as it nears the top floor or as it begins to descend to a lower floor, the acceleration is directed downward ($a \lt 0$). In these instances, the person riding the elevator feels lighter than normal.
If the elevator were to fall freely, with the acceleration due to gravity $a = -g$, you would calculate the normal force as \begin{align} N &= F_g + m a \nonumber\\[6pt] &= (m g) + m\, (-g) \nonumber\\[6pt] &= 0 \end{align} A person riding the elevator in this situation would feel weightless! This is actually how zero gravity is simulated on Earth. In what is popularly known as the “vomit comet,” astronaut trainees ride a special aircraft designed to fall down in a regulated manner for brief periods of time.
Video 9.1: Concept of gravity.
Questions
The following questions are selected from the end of Chapter 9 of the eText. It is important to your learning that you try to answer each question independently before you read through the answer and explanation given.
For questions that ask you to explain, defend, or discuss your answer, the response revealed by the Answer button would earn you only partial marks on a quiz or exam in this course. Use the Answer to help you formulate a complete answer before you select the Explanation button to check your work.
Note that some questions have been adapted to suit the format of this course.
Chapter 9
Question 12
When you weigh yourself on a bathroom scale in an elevator moving upward at constant velocity, are the springs in the scale more, or less, compressed? Moving downward at constant velocity?
Answer
There is no change in the compression of the springs inside the bathroom scale in either scenario.
Explanation
While moving at constant velocity (either upward or downward), the elevator system experiences no acceleration. This means that while standing on a bathroom scale inside the elevator, your acceleration (as well as the net force on your body) is equal to zero. Therefore, you conclude that the downward force of gravity (or your weight) is balanced by the upward normal (or support) force exerted by the bathroom scale on your feet. Since the scale is sensitive to the normal force, the measured weight would be equal to your true weight.
Chapter 9
Question 16
Do tides depend more on the strength of gravitational pull or on the difference in strengths? Explain.
Answer
Tides depend more on the difference in strength of gravitational pull.
Explanation
The gravitational pull of the Sun on Earth is 180 times greater than the gravitational pull of the Moon on Earth. Nevertheless, the ocean tides are caused mostly by the gravitational influence of the Moon. This is because the tidal effect depends on the difference in gravitational pull on opposite sides of Earth. Such difference in the lunar field is 2.2 times greater than the corresponding difference in the solar field.
Chapter 9
Question 33
\begin{equation} F = G\, \frac{m_1\, m_2}{r^2} \end{equation}
Calculate the force of Earth’s gravity on a 1-kg mass at Earth’s surface. The mass of Earth is $6.0\times 10^{24}\,\text{kg}$ and its radius is $6.4 \times 10^6\,\text{m}$. Does the result surprise you?
Answer
9.8 N
Explanation
To calculate the force of gravity on a body of mass $m = 1\,\text{kg}$ at Earth’s surface, substitute into the universal law of gravity, where $M_\text{E} = 6.0\times 10^{24}\,\text{kg}$ and $R_\text{E} = 6.4 \times 10^6\,\text{m}$, as follows: \begin{align} F_g &= G\, \frac{m M_\text{E}}{R_\text{E}^2} \nonumber\\[6pt] &= (6.67 \times 10^{-11}\, \text{N}\cdot\text{m}^2/\text{kg}^2)\times \frac{(1\,\text{kg}) (6.0\times 10^{24}\,\text{kg})}{(6.4 \times 10^6\,\text{m})^2} \nonumber\\[6pt] &= 9.8\,\text{N} \end{align} As expected, this is equal to the weight of a 1-kg object near Earth’s surface, where you have \begin{align} \text{Weight} &= m g \nonumber\\[6pt] &= (1\,\text{kg}) (9.8\,\text{m}/\text{s}^2) \nonumber\\[6pt] &= 9.8\,\text{N} \end{align} Note that in other questions in this course, the approximate value of $10\,\text{m}/\text{s}^2$ is used for the acceleration due to gravity.
Chapter 9
Question 34
\begin{equation} F = G\, \frac{m_1\, m_2}{r^2} \end{equation}
Calculate the force of gravity on the same 1-kg mass if it is $6.4 \times 10^6\,\text{m}$ above Earth’s surface (that is, if it is two Earth radii from Earth’s center).
Answer
$2.45\,\text{N}$
Explanation
You can calculate the force of gravity on a 1-kg mass located at two Earth radii from Earth’s center by using a calculation similar to the one in the answer to the previous question: \begin{align} F_g &= G\, \frac{m M_\text{E}}{(2 R_\text{E})^2} \nonumber\\[6pt] &= \frac{1}{4} \left(G\, \frac{m M_\text{E}}{R_\text{E}^2}\right) \nonumber\\[6pt] &= \frac{9.8\,\text{N}}{4} \nonumber\\[6pt] &= 2.45\,\text{N} \end{align} Note that this value is one-quarter of the value you obtained in the answer to the previous question. This is because the force of gravity decreases by four times when the distance doubles.
Chapter 9
Question 39
Show that there is no change in the force of gravity between two objects when their masses are doubled and the distance between them is also doubled.
Answer
Starting with the gravitational force equation \begin{equation} F_1 = G\, \frac{m_1\, m_2}{r^2} \end{equation} when you double the masses of the two objects ($m_1$ and $m_2$) and also the distance ($r$) between them, you can write \begin{align} F_2 &= G\, \frac{(2 m_1) (2 m_2)}{(2r)^2} \nonumber\\[6pt] &= G\, \frac{4\, m_1\, m_2}{4\, r^2} \nonumber\\[6pt] &= G\, \frac{m_1\, m_2}{r^2} \nonumber\\[6pt] &= F_1 \end{align} You see that doubling each mass contributes a factor of 2, while doubling the distance contributes a factor of 1⁄4.
Chapter 9
Question 47
Pretend you fall into a hole bored completely through the Earth, with no obstructions along the way. Rank positions A, B, C, and D from most to least for your
- speed.
- acceleration toward Earth’s center.
Figure Q9.47
Answer
- $\text{B}$, $\text{A} = \text{C}$, then $\text{D}$
- $\text{D}$, $\text{A} = \text{C}$, then $\text{B}$
Explanation
- The gravitational force below the ground pulls your body toward Earth’s center, and the longer it pulls on you, the more speed you gain. Hence, the greatest speed is attained at position B (see Figure Q9.47), Earth’s center. After passing position B, gravity acts to pull you back toward Earth’s center, thus slowing you down until you stop completely when you reach position D. So the further away you are from the center, the slower your speed is.
- The gravitational force inside Earth decreases as you approach the center (see Figure 9.24 in the eText). Therefore, your acceleration is greatest near Earth’s surface and decreases as you fall deeper into the hole, until it becomes zero at the center.
Chapter 9
Question 67
Is the acceleration due to gravity more or less atop Mt. Everest than at sea level? Defend your answer.
Answer
The acceleration due to gravity is less atop Mt. Everest than at sea level. The force of gravity (and consequently the acceleration due to gravity) decreases with increasing distance from Earth’s center. Since the peak of Mt. Everest is nearly 9 km above sea level, the acceleration due to gravity decreases as you climb the mountain. When you calculate the acceleration due to gravity ($g$), you find that it drops by approximately 0.3% at the top of the mountain.
Chapter 9
Question 70
Why does a person in free fall experience weightlessness, while a person falling at terminal velocity does not?
Answer
Apparent weight is equal to the support force, which is absent during free fall, and equal to $mg$ while an object is falling at terminal velocity.
Explanation
A person falling freely (in the absence of air resistance) does not experience any upward force; therefore, their body is not pressed against any object to create a sense of weight. As a result, the person feels weightless in this situation. However, when a person is falling with constant terminal velocity, the net force on the person is equal to zero. In this case, air drag provides an upward support force equal to the person’s weight and creates a sense of weight.
Chapter 9
Question 72
Is gravitational force acting on you when soaring across the ground in your jetsuit? On an astronaut inside an orbiting space shuttle? Defend your answer.
Answer
Yes, in both situations.
Explanation
Gravitational forces always exist between objects regardless of whether they are in motion or at rest. Therefore, the force of gravity between your body (in jetsuit) and Earth and between an astronaut (in a space shuttle) and Earth cannot be turned off or shielded by any material or vacuum.
Chapter 9
Question 88
Why aren’t high ocean tides exactly 12 hours apart?
Answer
Because the lunar day is longer than the solar day.
Diagram
Video Q9.88
Explanation
While Earth spins about its axis, the Moon advances in its orbit around Earth. Therefore, a specific location on Earth directly facing the Moon will take a full lunar day (24 hours and 50 minutes) for the next alignment with the Moon (see Video Q9.88). As a result, high ocean tides occur 12 hours and 25 minutes apart.
Chapter 9
Question 99
A friend says that above the atmosphere, in space shuttle territory, Earth’s gravitational field is zero. Discuss your friend’s misconception by using the equation for gravitational force in your explanation.
Answer
It is true that the atmosphere is held around Earth by gravity and that air density decreases with altitude and practically disappears after a few hundred kilometers. However, the force of gravity does not vanish past the atmosphere. The gravitational force equation shows that the force exerted by Earth on a space shuttle diminishes as the shuttle travels farther from Earth, but $F$ never becomes zero. For example, at an altitude of 500 km, the force of gravity on the space shuttle is only 14% less than what it is on the ground a the Kennedy Space Center.
Note that an orbiting space shuttle moves around Earth along a circular orbit, where the force of gravity provides the required centripetal force.
Chapter 9
Question 100
Somewhere between Earth and the Moon, gravity from these two bodies on a space pod would cancel. Is this location nearer Earth or the Moon? Why?
Answer
This location is much closer to the Moon.
Diagram
Figure Q9.100
Explanation
Earth is 81 times more massive than the Moon. Therefore, a space pod located midway between Earth and the Moon experiences a pull toward Earth that is 81 times stronger than that toward the Moon.
The force of gravity, however, is also inversely proportional to the square of the distance. So if the space pod moves closer to the Moon such that the distance to Earth ($d_\text{E}$) is nine times the distance to the Moon ($d_\text{M}$), the pod will experience equal forces from the two bodies, resulting in a zero net force (see Figure Q9.100). In this case, the factor of 81 due to the larger mass is canceled by a factor of $1/9^2 = 1/81$ due to the greater distance.
Mathematically speaking, when $M_\text{E} = 81\, M_\text{M}$ and $d_\text{E} = 9\, d_\text{M}$, the force of gravity on the pod (of mass $m$) due to the Moon is given by \begin{equation} F_\text{M} = G\, \frac{m\, M_\text{M}}{d_\text{M}^2} \end{equation} In this case, the gravitational force applied by Earth is \begin{align} F_\text{E} &= G\, \frac{m\, M_\text{E}}{d_\text{E}^2} \nonumber\\[6pt] &= G\,\frac{m\, (81\, M_\text{M})}{(9\, d_\text{M})^2} \nonumber\\[6pt] &= G\,\frac{m\, (81\, M_\text{M})}{81\, d_\text{M}^2} \nonumber\\[6pt] &= G\,\frac{m\, M_\text{M}}{d_\text{M}^2} \nonumber\\[6pt] &= F_\text{M} \end{align}
Chapter 9
Question 103
The planet Jupiter is more than 300 times as massive as Earth, so it might seem that a body on the surface of Jupiter would weigh 300 times as much as on Earth. But a body would weigh scarcely 3 times as much on the surface of Jupiter as it would on the surface of Earth. Discuss why using the terms in the equation for gravitational force to guide your thinking.
Hint
The increase in the body’s weight due to Jupiter’s mass is partially offset by Jupiter’s size.
Diagram
Figure Q9.103
Answer
The force of gravity exerted by a planet on a body is proportional to its mass and inversely proportional to the square of the distance to the planet’s center. If Jupiter were the same size as Earth, a body near the surface of Jupiter would weigh 300 times more than on Earth’s surface. This is because Jupiter is 300 times more massive than Earth. The radius of Jupiter, however, is roughly 10 times that of Earth (see Figure Q9.103). This reduces the gravitational attraction by a factor of $10^2 = 100$ on the surface of the giant planet. When both factors are combined, the net increase in the body’s weight is only by a factor of $300\times 1/100 = 3$.
Chapter 9
Question 105
Explain why this reasoning is wrong: “The Sun attracts all bodies on Earth. At midnight, when the Sun is directly below, it pulls on you in the same direction as Earth pulls on you; at noon, when the Sun is directly overhead, it pulls on you in a direction opposite to Earth’s pull on you. Therefore, you should be somewhat heavier at midnight and somewhat lighter at noon.”
Answer
All objects on the Earth are in a state of continuous free fall around the Sun.
While orbiting the Sun, the entire Earth system experiences an acceleration due to solar gravity that is approximately equal to $0.006\,\text{m}/\text{s}^2$. This is also equal to the centripetal acceleration directed toward the Sun, which every object on Earth experiences at all times.
So when you stand on a bathroom scale, both you and the scale rotate around the Sun with the same speed and acceleration. As a result, the Sun’s gravitational attraction does not contribute to the contact force between your feet and the scale. In other words, the rotation around the Sun does not cause your feet to press harder (or less hard) on the scale, which in turn does not change the support force it exerts on your feet. Therefore, you should not feel any change in your weight between noon and midnight due to the position of the Sun.
Chapter 9
Question 108
Does the fact that one side of the Moon always faces Earth mean that the Moon rotates about its axis (like a top) or that it doesn’t rotate about its axis? Discuss and defend your answer.
Diagram
Video Q9.108
Answer
The Moon does rotate about its axis, and its spin about its axis is synchronized with its rotation around Earth. This means that the time it takes the Moon to complete one rotation about its axis is equal to the period of its complete revolution around Earth. In Video Q9.108 (not drawn to scale), you can see how the Moon spins slowly about its axis such that only one side faces Earth all the time. Note that if the Moon did not rotate about its axis, you would see all parts of the Moon’s surface during a lunar month.
Additional Question
Answer the following additional question (not found in your eText).
Unit 9
Question A
Explain why all freely falling bodies experience the same acceleration regardless of their masses.
Answer
The force of gravity on a body of mass $m$ is given by the equation
\begin{equation} F_g = G\, \frac{m\, M_\text{E}}{r^2} \label{u09_AQA_Fg} \end{equation}
where $M_\text{E}$ is the mass of Earth and $r$ is the distance between the body and the center of Earth. According to Newton’s second law, the acceleration of a body under the influence of gravity only is given by
\begin{equation} a = \frac{F_g}{m} \label{u09_AQA_aFm} \end{equation}
By substituting from Equation 9.16 into Equation 9.17, you have
\begin{align} a &= \left(G\, \frac{m\, M_\text{E}}{d^2}\right) \times \frac{1}{m} \nonumber\\[6pt] &= G\, \frac{M_\text{E}}{d^2} \label{u09_AQA_aQMEd}\textrm{.} \end{align}
From Equation 9.18, you can see that the acceleration of a freely falling body is independent of its mass.
Exercises
Spend some time completing the following exercises to test your understanding of the main concepts in Chapter 9 and increase your efficiency in answering exam questions.
End-of-Chapter Practice Questions
Answer questions 3, 11, 13, 17, 23, 27, 29, 35, 43, 45, 55, 59, 61, 69, 79, 85, 93, 101, 109, and 115 from Chapter 9 of the eText. If you require assistance, please contact your tutor. The answers are provided at the end of the eText.